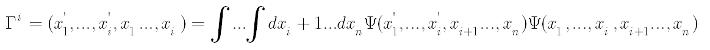The electronic wave function is the ultimate source of information that determines all (electronic) properties of a molecule. For practical calculations, however, it is a far too complicated object to manipulate. Fortunately, most of the relevant information can be condensed into so-called density matrices, which have a lower complexity.

The single-particle density matrix, e.g., lies at the basis of the natural spinorbitals, a popular concept in quantum chemistry.

Concepts such as atoms and functional groups are absent in the quantum physical approach to molecules. Yet these "information shortcuts" have proven to be extremely successful in the development of modern chemistry. Chemical reactivity is understood in
terms of atomic charges, binding energies, nucleophile and electrophile functional groups, electron withdrawing or donating groups, basic and acidic groups,... A quantum physical definition of atoms and functional groups would allow to quantify and catalogue these chemical characteristics in an unambiguous and simple way.

Up to the present such quantum physical "atoms in molecules" (AIM) have been constructed at the level of the electronic density (which is the diagonal of the single-particle density matrix in coordinate space). Depending on the definition used (Mulliken, Bader, Hirshfeld,...), sometimes very dissimilar results were obtained for AIM properties like the effective charges.

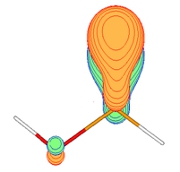
Fig 1: Natural orbital in COOH : the carbonyl pi-bond.

A hot topic in quantum chemistry is therefore the extension of quantum physical AIM's to the level of the single-particle density matrix [1]. This provides essential information on how a molecular orbital is formed out of atomic ones. A very recent development is to apply AIM partitioning techniques to the difference of neutral and ionic molecular density matrices. These are the so-called Fukui matrices which provide information on the "easiness" to attach or remove an additional electron in selected molecular regions. Up to now, this has only been done at the level of self-consistent field (Hartree-Fock, Density Functional) theory. This topic therefore allows many angles: to generalize the method to a correlated (post-HF) description of the molecule, or to collaborate on the development of a new AIM partitioning algorithm.The thesis work involves therefore the creation and implementation of partitioning algorithms using molecular modelling software, and the numerical testing of the algorithm.